Rene Descartes

Rene Descartes was a very important and famous philosopher of the 17th century. He mostly focused on life in general, but was indeed very important to modern-day mathematics, especially geometry.

In 1637 he published his world changing book called "Discourse on Method". This book was extremely important to algebra (and of course geometry, since algebra and geometry are majorly related) because he began using standard lowercase notations such as: a, b, c for known quantities and x, y, z for unknown quantities. This was an incredible "invention" for even today, almost 380 years later, we still use it in everyday geometry and overall math.

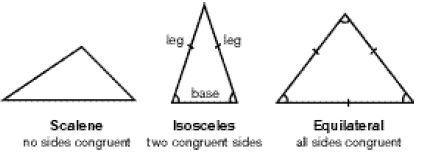
Later he also stated that each point in a 2D plane, can be described as two numbers; one showing horizontal location and the other one describing vertical location. He used perpendicular lines to discover the point's x (horizontal) and y (vertical) coordinates. This can be seen on the figure below:

       

He was also able to create "a rule of signs" technique which many stated to be his most important contribution to geometry as we know it. This technique determined the number of positive and/or negative (real) roots of a polynomial. He also popularised the notion of exponents throughout major parts of Europe.



He was an incredible philosopher, a great physician, but most importantly; he was an outstanding mathematician which shaped math itself. Some may even consider him one of the funding fathers of geometry!         

Midpoint Formula

Midpoint Formula [mid-point fawr-myuh-luh]
Noun
The midpoint formula is practically the averages of both the x-coordinate and the y-coordinate. This simple, yet useful formula is:

   

It's extremely useful in geometry when you need to find a point that is between two other points. It may and should be applied when trying to figure out a line that bisects a specific line segment. It's is

used all over geometry especially when trying to find the midpoint (therefore the name: midpoint formula) of a line segment.  

   

Parallel lines theorem and Perpendicular lines thereom

Parallel lines theorem [par-uh-lel lahyns thee-er-uhm]
Two lines are parallel if they're intersected by a transversal in such way that:

• The corresponding angles are congruent.
• The alternate interior angles are congruent.
• The alternate exterior angles are congruent.
• The same-side interior angles are supplementary (have a sum of 180 degrees).
• The same-side exterior angles are supplementary (have a sum of 180 degrees).

Perpendicular lines theorem [pur-puh-n-dik-yuh-ler lahyns thee-er-uhm]
Given a line (line 1) and a point that's not located on that exact line, there is one and only one line through the given point that is perpendicular to the given line (line 1).

Slope

Slope [slohp]
Noun
Slope in mathematics describes the steepness (degree) of a line. Also in mathematics, slopes only go from left to right, unlike slopes in real life. A slope can be negative, positive or zero.
Example:

 Positive Slope:

  

Negative Slope:

Zero Slope (practically, no slope at all) 

There are three main steps in finding the slope of a straight line:

1) Identify any of the line's two points 

2) Choose which one will be (x1,y1) and (x2,y2)

3) Use the equation located on the bottom do find the slope of the line

Why is the midsegment of a triangle and a trapezoid important?

Before we talk on how a triangle's midsegment is important, we have to talk on how it's found. Well, to find the midsegment of a triangle, you have to find the midpoints of two of the triangle's side. Once you have found the two midpoints, you will connect them both with a line. That's it! You've just found the triangle's midsegment! One important thing to note is that the midsegment will always be parallel to the third side (the one you didn't connect midpoints with). So in total you can find three midsegments. What's very important about the midsegments, is that they will always be half the length of the third segment (the one you didn't connect midpoints with). To summarise, a triangle's midsegments are very useful to find the triangles side's length.  

Note: on the triangle on top, the segment DE is half (length) of segment BC.

Useful Video

The midsegment on a trapezoid is found the same way as the triangle, but it will be parallel to the two remaining bases. Also it has the average length of both of the trapezoid's bases.  The midsegment on a trapezoid divides it into two smaller trapezoids. This is important for each of the trapezoids will have half the altitude of the original one. With that in mind you will use the midsegment's length times the trapezoid's total altitude to find its area.      

Useful Link

How do the sides of a polygon relate to its interior angle's degrees?

This method I'm going to tell you only works on regular polygons (all sides are congruent)! It's a method which will tell you the degree of each interior angle of the polygon. Since regular polygons all have congruent sides, all angles will have the same degree. Does not work with irregular polygons!
It's a very simple method, you really just need to know how many angles/sides the polygon has. The equation is 180(n-2)/n. 
n= number of angles/sides the polygon has
Example:

This is a regular hexagon. A hexagon has 6 sides and 6 angles. Therefore we plug 6 as n.

180(6-2)/6

180(4)/6

720/6

120

Each of a regular hexagon's interior angles have a measurement of 120 degrees.

You will later notice (after trying out different regular polygon) that the more sides and angle a regular polygon has, the more degrees its interior angles will have. 

Example:

  Regular hexagons angle's measurement: 120 degrees

Regular pentagons angle's measurement: 108 degrees

 
Squares angle's measurement: 90 degrees

Equilateral triangles angle's measurement: 60 degrees 

Triangle Sum Theorem

Triangle Sum Theorem [trahy-ang-guhl suhm theer-uhm]
Noun
This theorem clearly states that the sum of any triangle's (equilateral, isosceles, scalene, etc.) interior angles have to equal 180 degrees.
A nice two-column proof of this theorem is:  

Statements	Reasons
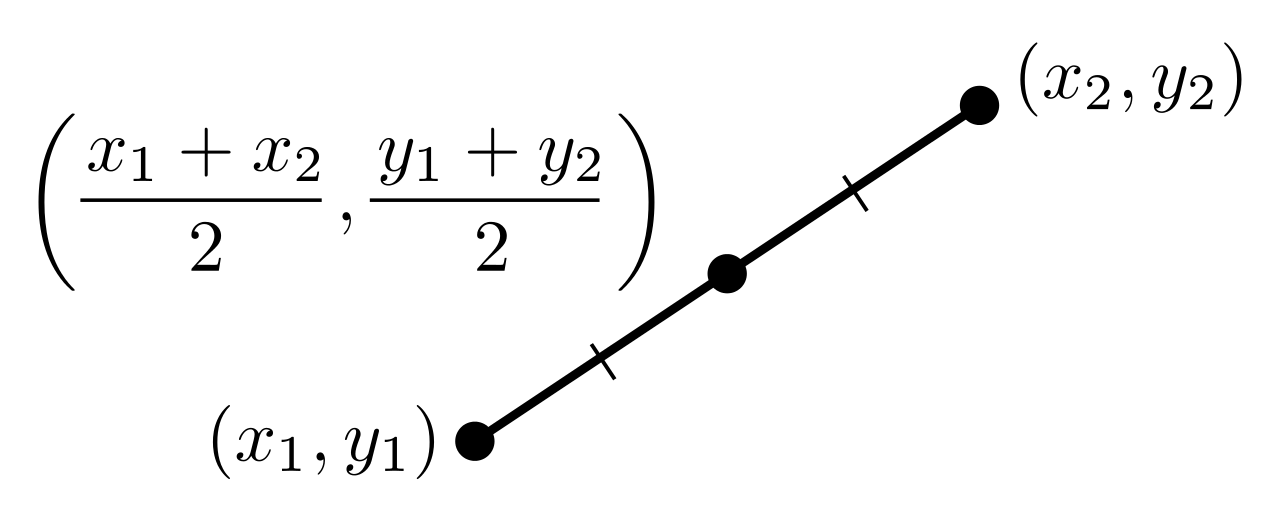
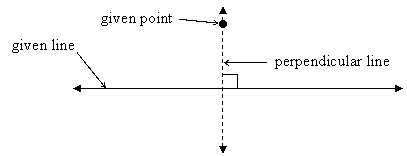
Construct a line parallel to through point Y. Call this line	Construction
m1 + m5 = mAYX	Angle Addition Postulate
mAYX + m4 = 180°	Linear Pair Postulate
m1 + m5 + m4 = 180°	Substitution
	Alternate Interior Angles Theorem
m2 = m4 m3 = m5	Definition of Congruence
m1 + m3 + m2 = 180°	Substitution

URL

How can one prove that two lines are parallel?

There are four major ways of proving if two lines are parallel.

1. Corresponding Angles Postulate

If you read the previous post then you already know that corresponding angles are angles which are formed when a transversal crosses two other lines. 

The following are corresponding angles:

1 and 5

2 and 6

3 and 7

4 and 8

Knowing corresponding angles is very useful to make sure two angles are parallel, for if the corresponding angles have the same measurement (degree), then the two lines being crossed by the transversal are parallel. If their measurement are different then, no, they are not parallel.   

2.  Alternate Interior Angles Theorem

3. Alternate Exterior Angles Theorem  
    

The following are alternate exterior angles: 

8 and 1

7 and 2

The following are alternate interior angles:

6 and 3

5 and 4 

The previous post also talked about alternate interior and exterior angles theorem. This is useful to figure out if two lines are parallel because if the alternate interior angles are congruent (have the same measurement), then the two lines are automatically parallel. Same thing goes with the alternate exterior angles; if they are congruent, then the two lines being intersected by the transversal are parallel. 

4. Consecutive Interior Angles Theorem 

Consecutive angles, are angles which lay on the same side of the transversal and are on the inside of the two lines.

The following are consecutive interior angles: 

6 and 4

3 and 5

This theorem is useful to find out if two lines are parallel because if both the consecutive interior angles' measurement add up to 180, then the two lines are parallel.  

Corresponding Angles

Corresponding Angles [kawr-uh-spon-ding ang-guhls]
Noun
Corresponding angles are, well, angles which are created when a transversal crosses/intersects two other lines. When this intersection occurs, the matching angle corners are considered to be corresponding each other, therefore the name.
There is a theorem which states that: if the two lines being intersected by the transversal are parallel, then the corresponding angles will be congruent. In the figure below you can clearly see this theorem at work.
There also exists alternate exterior angles and interior angles. Alternate exterior angles are each on the opposite side of the transversal and are on the outside of the two lines. Just like the exterior angles, the alternate interior angles are on the opposite side of the transversal, but unlike the exterior, they're on the inside of the two lines.  
 

t=transversal 

 

The following are corresponding angles:

1 and 5

2 and 6

3 and 7

4 and 8
The following are alternate exterior angles: 
8 and 1
7 and 2
The following are alternate interior angles:
6 and 3
5 and 4 

Transversal

Transversal [trans-vur-suhl]
Noun
A transversal is a line that crosses/intersects two or more lines, therefore if it only intersects one line it is not a transversal.

Quadrilateral

Quadrilateral [kwod-ruh-lat-er-uhl]
Noun
A quadrilateral is any polygon which has four straight sides. Doesn't have to be four equal sides though. In the name it clearly shows its definition; quad meaning four and lateral meaning sides. There are many different types of quadrilateral, but the most famous ones are:

  

Rotational Symmetry

Rotational Symmetry [roh-tey-shuh-nal sim-i-tree]
Noun
Rotational Symmetry is when you rotate a image/shape and yet it does not become distorted, therefore it retains its shape. How many matches (shape looks exactly the same when rotated) there are when turning the figure, is called the order. Depending on the figure it can have an order of 2, 5, 10 etc.

This dartboard has an order of 10

This star has an order of 5

Triangle Classification

Triangle [trahy-ang-guh-l]
Noun
A triangle is a 2D closed figure which has exactly 3 angles and three sides (tri-angle)

Triangles are usually classified by the length of their sides (they might also be classified by their angles).

There are three major classification of triangles:

  

Reflection Symmetry

Reflectional Symmetry [Ree-fle-ction-nal Sim-i-tree] 

Noun

Reflectional Symmetry is very easy to spot in nature and in our surroundings. Sometimes called Line Symmetry, it is so simple to spot for one half is the exact reflection of the other. The line of symmetry can either run vertically or horizontally.

Example: The orchard's reflection on the nearby lake (horizontal line of symmetry)

    Horizontal line of symmetry 

Vertical line of symmetry 

Polygon

Polygon [pol-ee-gon]

Noun

A polygon is a 2D plane which have straight sides. There are two main type of polygons; irregular and regular. Regular polygons' sides are all equal, while the irregular polygons' sides aren't all equal/ congruent. 

 Not a polygon for it contains a curved side

Not a polygon for it's not a closed figure

Is a polygon for it's closed and it has straight sides

The sides of a Polygon are segments which closes the figure making it a Plane. The point where the line segments meet are called vertex (plural: vertices)